LeetCode - Path Sum II
Problem statement
Given the root of a binary tree and an integer targetSum, return all root-to-leaf paths where the sum of the node values in the path equals targetSum. Each path should be returned as a list of the node values, not node references.
A root-to-leaf path is a path starting from the root and ending at any leaf node. A leaf is a node with no children.
Problem statement taken from: https://leetcode.com/problems/path-sum-ii
Example 1:
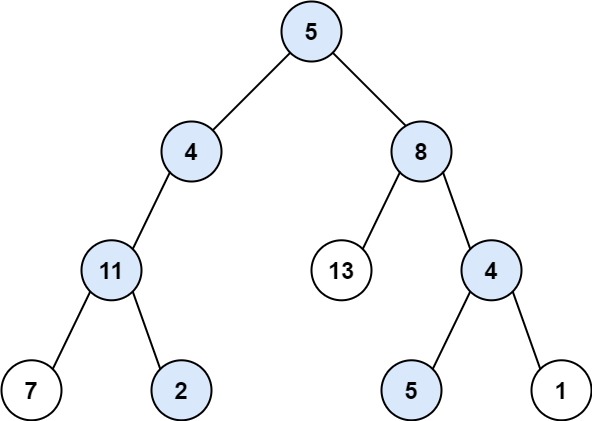
Input: root = [5, 4, 8, 11, null, 13, 4, 7, 2, null, null, 5, 1], targetSum = 22
Output: [[5, 4, 11, 2], [5, 8, 4, 5]]
Explanation: There are two paths whose sum equals targetSum:
5 + 4 + 11 + 2 = 22
5 + 8 + 4 + 5 = 22
Example 2:
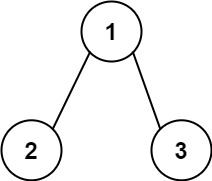
Input: root = [1, 2, 3], targetSum = 5
Output: []
Example 3:
Input: root = [1, 2], targetSum = 0
Output: []
Constraints:
- The number of nodes in the tree is in the range [0, 5000]
- -1000 <= Node.val <= 1000
- -1000 <= targetSum <= 1000
Explanation
The problem is similar to our previous blog post Path Sum. We will use the same algorithm flow here, but we will also store the tree node values in an array.
Let's check the algorithm first.
- set 2D array vector<vector<int>> result
1D array vector<int> current
- getPathSum(root, result, current, targetSum)
- return result
// getPathSum function
- if root == NULL
- return
- if root->val == targetSum && root->left == NULL && root->right == NULL
- current.push_back(root->val)
- result.push_back(current)
- return
- set remainingTargetSum = targetSum - root->val
- current.push_back(root->val)
- getPathSum(root->left, result, current, remainingTargetSum)
- getPathSum(root->right, result, current, remainingTargetSum)
- return
Let's check our algorithm in C++, Golang, and Javascript.
C++ solution
class Solution {
public:
void getPathSum(TreeNode* root, vector<vector<int>> &result, vector<int> current, int targetSum) {
if(root == NULL) {
return;
}
if(root->val == targetSum && root->left == NULL && root->right == NULL) {
current.push_back(root->val);
result.push_back(current);
return;
}
int remainingTargetSum = targetSum - root->val;
current.push_back(root->val);
getPathSum(root->left, result, current, remainingTargetSum);
getPathSum(root->right, result, current, remainingTargetSum);
return;
}
vector<vector<int>> pathSum(TreeNode* root, int targetSum) {
vector<vector<int>> result;
vector<int> current;
getPathSum(root, result, current, targetSum);
return result;
}
};Golang solution
func getPathSum(root *TreeNode, result *[][]int, current []int, targetSum int) {
if root == nil {
return
}
if root.Val == targetSum && root.Left == nil && root.Right == nil {
current = append(current, root.Val)
*result = append(*result, append([]int(nil),current...))
return
}
remainingTargetSum := targetSum - root.Val
current = append(current, root.Val)
getPathSum(root.Left, result, current, remainingTargetSum)
getPathSum(root.Right, result, current, remainingTargetSum)
return
}
func pathSum(root *TreeNode, targetSum int) [][]int {
result := [][]int{}
current := []int{}
getPathSum(root, &result, current, targetSum)
return result
}Javascript solution
var pathSum = function(root, targetSum) {
let result = [];
var getPathSum = function(root, current, targetSum) {
if(root === null) {
return;
}
if(root.val === targetSum && root.left === null && root.right === null) {
result.push([...current, root.val]);
return;
}
let remainingTargetSum = targetSum - root.val;
getPathSum(root.left, [...current, root.val], remainingTargetSum);
getPathSum(root.right, [...current, root.val], remainingTargetSum);
return;
}
getPathSum(root, [], targetSum);
return result;
};Dry Run
Let's dry-run our algorithm for Example 1.
Input: root = [5, 4, 8, 11, null, 13, 4, 7, 2, null, null, 5, 1]
targetSum = 22
Step 1: initialize vector<vector<int>> result;
vector<int> current;
Step 2: getPathSum(root, result, current, targetSum)
getPathSum(root, [[]], [], 22)
the root is at 5
// getPathSum
Step 3: if root == NULL
false
if root->val == targetSum && root->left == NULL && root->right == NULL
5 == 22
false
remainingTargetSum = targetSum - root->val
= 22 - 5
= 17
current.push_back(root->val)
current = [5]
getPathSum(root->left, result, current, remainingTargetSum)
getPathSum(4, [[]], [5], 17)
Step 4: if root == NULL
the root is at 4
false
if root->val == targetSum && root->left == NULL && root->right == NULL
4 == 17
false
remainingTargetSum = targetSum - root->val
= 17 - 4
= 13
current.push_back(root->val)
current = [5, 4]
getPathSum(root->left, result, current, remainingTargetSum)
getPathSum(11, [[]], [5, 4], 13)
Step 5: if root == NULL
the root is at 11
false
if root->val == targetSum && root->left == NULL && root->right == NULL
11 == 13
false
remainingTargetSum = targetSum - root->val
= 13 - 11
= 2
current.push_back(root->val)
current = [5, 4, 11]
getPathSum(root->left, result, current, remainingTargetSum)
getPathSum(7, [[]], [5, 4, 11], 2)
Step 6: if root == NULL
the root is at 7
false
if root->val == targetSum && root->left == NULL && root->right == NULL
7 == 2
false
remainingTargetSum = targetSum - root->val
= 2 - 7
= -5
current.push_back(root->val)
current = [5, 4, 11, 7]
getPathSum(root->left, result, current, remainingTargetSum)
getPathSum(NULL, [[]], [5, 4, 11, 7], -5)
Step 7: if root == NULL
the root is NULL
true
We backtrack to step 6 and continue
Step 8: getPathSum(root->right, result, current, remainingTargetSum)
getPathSum(2, [[]], [5, 4, 11], 2)
Step 9: if root == NULL
the root is at 2
false
if root->val == targetSum && root->left == NULL && root->right == NULL
2 == 2 && root->left == NULL && root->right == NULL
true
current.push_back(root->val)
current = [5, 4, 11, 2]
result.push_back(current)
result = [[5, 4, 11, 2]]
return
We backtrack to step 4 and continue
Step 10: getPathSum(root->right, result, current, remainingTargetSum)
getPathSum(NULL, [[5, 4, 11, 2]], [5, 4], 13)
Step 11: if root == NULL
the root is NULL
true
We backtrack to step 3 and continue
Step 12: getPathSum(root->right, result, current, remainingTargetSum)
getPathSum(8, [[5, 4, 11, 2]], [5], 17)
Step 13: if root == NULL
the root is at 8
false
if root->val == targetSum && root->left == NULL && root->right == NULL
8 == 17
false
remainingTargetSum = targetSum - root->val
= 17 - 8
= 9
current.push_back(root->val)
current = [5, 8]
getPathSum(root->left, result, current, remainingTargetSum)
getPathSum(13, [[5, 4, 11, 2]], [5, 8], 9)
Step 14: if root == NULL
the root is at 13
false
if root->val == targetSum && root->left == NULL && root->right == NULL
13 == 9
false
remainingTargetSum = targetSum - root->val
= 9 - 13
= -4
current.push_back(root->val)
current = [5, 8, 13]
getPathSum(root->left, result, current, remainingTargetSum)
getPathSum(NULL, [[5, 4, 11, 2]], [5, 8, 13], -4)
Step 15: if root == NULL
the root is NULL
true
We backtrack to step 14 and continue
Step 16: getPathSum(root->right, result, current, remainingTargetSum)
getPathSum(NULL, [[5, 4, 11, 2]], [5, 8, 13], -4)
Step 17: if root == NULL
the root is NULL
true
We backtrack to step 13 and continue
Step 18: getPathSum(root->right, result, current, remainingTargetSum)
getPathSum(4, [[5, 4, 11, 2]], [5, 8], 9)
Step 19: if root == NULL
the root is at 4
false
if root->val == targetSum && root->left == NULL && root->right == NULL
4 == 9
false
remainingTargetSum = targetSum - root->val
= 9 - 4
= 5
current.push_back(root->val)
current = [5, 8, 4]
getPathSum(root->left, result, current, remainingTargetSum)
getPathSum(5, [[5, 4, 11, 2]], [5, 8, 4], 5)
Step 20: if root == NULL
the root is at 5
false
if root->val == targetSum && root->left == NULL && root->right == NULL
5 == 5
true
current.push_back(root->val)
current = [5, 8, 4, 5]
result.push_back(current)
result = [[5, 4, 11, 2], [5, 8, 4, 5]]
return
We backtrack to step 19 and continue
Once the last rightmost lead node is processed
we return the result
The answer is [[5, 4, 11, 2], [5, 8, 4, 5]].