LeetCode - House Robber III
Problem statement
The thief has found himself a new place for his thievery again. There is only one entrance to this area, called root.
Besides the root, each house has one and only one parent house. After a tour, the smart thief realized that all houses in this place form a binary tree. It will automatically contact the police if two directly-linked houses were broken into on the same night.
Given the root of the binary tree, return the maximum amount of money the thief can rob without alerting the police.
Problem statement taken from: https://leetcode.com/problems/house-robber-iii/
Example 1:
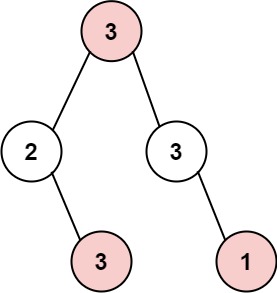
Input: root = [3, 2, 3, null, 3, null, 1]
Output: 7
Explanation: Maximum amount of money the thief can rob = 3 + 3 + 1 = 7.
Example 2:
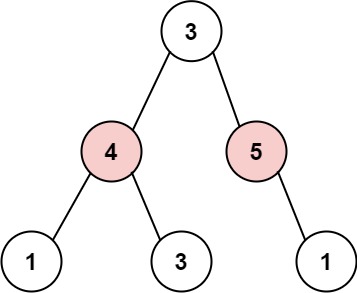
Input: root = [3, 4, 5, 1, 3, null, 1]
Output: 9
Explanation: Maximum amount of money the thief can rob = 4 + 5 = 9.
Constraints:
- The number of nodes in the tree is in the range [1, 10^4].
- 0 <= Node.val <= 10^4
Explanation
This problem is similar to our previous two blog posts House Robber and House Robber II. The houses are arranged in a binary tree fashion, and they are neither in a straight line nor in a circle.
We can use the Dynamic Programming approach here. We need to change how our DP array will be set and consumed. The problem approach should be:
We create a helper function to traverse the tree from the root.
Recursively travel the root's left and right subtree.
Two directly-linked houses should be robbed. If the root node is considered, we should consider the grandchild nodes and leave out the child nodes.
If the root is excluded, and we consider the child nodes, then leave its immediate child nodes and consider its grandchild nodes.
Return the result as the maximum of root and its grandchild or the immediate child level summation.
Let's check the algorithm first.
// function rob(TreeNode* root)
- set array result = robHelper(root)
- return max(result[0], result[1])
// function robHelper(root)
- if root == NULL
- return [0, 0]
- initialize left and right array
- left = robHelper(root->left)
right = robHelper(root->right)
- totalIncludingRoot = root->val + left[1] + right[1]
totalExcludingRoot = max(left[0], left[1]) + max(right[0], right[1])
- return [totalIncludingRoot, totalExcludingRoot]
Let's check our solutions in C++, Golang, and Javascript.
C++ solution
class Solution {
public:
pair<int, int> robHelper(TreeNode* root) {
if(root == NULL){
return {0, 0};
}
pair<int, int> left, right;
left = robHelper(root->left);
right = robHelper(root->right);
int rootExcluded = max(left.first, left.second) + max(right.first, right.second);
int rootIncluded = root->val + left.second + right.second;
return {rootIncluded, rootExcluded};
}
int rob(TreeNode* root) {
pair<int, int> result = robHelper(root);
return max(result.first, result.second);
}
};Golang solution
func max(a, b int) int {
if a > b {
return a
}
return b
}
func robHelper(root *TreeNode) []int {
if root == nil {
return []int{0, 0}
}
left, right := robHelper(root.Left), robHelper(root.Right)
rootExcluded := max(left[0], left[1]) + max(right[0], right[1])
rootIncluded := root.Val + left[1] + right[1]
return []int{rootIncluded, rootExcluded}
}
func rob(root *TreeNode) int {
result := robHelper(root)
return max(result[0], result[1])
}Javascript solution
var robHelper = function(root) {
if(root === null) {
return [0, 0];
}
let left = robHelper(root.left);
let right = robHelper(root.right);
let rootExcluded = Math.max(left[0], left[1]) + Math.max(right[0], right[1]);
let rootIncluded = root.val + left[1] + right[1];
return [rootIncluded, rootExcluded];
}
var rob = function(root) {
let result = robHelper(root);
return Math.max(result[0], result[1]);
};Let's dry run our algorithm for a given input.
Input: root = [3, 2, 3, null, 3, null, 1]
// function rob(TreeNode* root)
Step 1: pair<int, int> result = robHelper(root)
// function robHelper(root)
Step 2: root == NULL
the root is pointing at 3
false
pair<int, int> left, right
left = robHelper(root->left)
root->left is pointing at 2
Step 3: root == NULL
the root is pointing at 2
false
pair<int, int> left, right
left = robHelper(root->left)
root->left is pointing at null
Step 4: root == NULL
the root is pointing at null
true
return [0, 0]
We backtrack to step 3 and move forward.
left = [0, 0]
right = robHelper(root->right)
the root is 2, and root->right is pointing to 3
Step 5: root == NULL
the root is pointing at 3
false
pair<int, int> left, right
left = robHelper(root->left)
root->left is pointing at null
Step 6: root == NULL
the root is pointing at null
true
return [0, 0]
We backtrack to step 5 and move forward.
left = [0, 0]
right = [0, 0]
the root is 3, and root->right is pointing to null
rootExcluded = Math.max(left[0], left[1]) + Math.max(right[0], right[1])
= Math.max(0, 0) + Math.max(0, 0)
= 0
rootIncluded = root.val + left[1] + right[1]
= 3 + 0 + 0
= 3
return [rootIncluded, rootExcluded]
[3, 0]
We backtrack to step 4
Step 7: left = [0, 0]
right = robHelper(root->right)
= [3, 0]
the root is 2, and root->right is pointing to 3
rootExcluded = Math.max(left[0], left[1]) + Math.max(right[0], right[1])
= Math.max(0, 0) + Math.max(3, 0)
= 3
rootIncluded = root.val + left[1] + right[1]
= 2 + 0 + 0
= 2
return [rootIncluded, rootExcluded]
[2, 3]
We backtrack to step 2, where the root is pointing to 3.
Step 8: left = robHelper(root->left)
= [2, 3]
right = robHelper(root->right)
root->right is pointing at 3
Step 9: root == NULL
the root is pointing at 3
false
pair<int, int> left, right
left = robHelper(root->left)
root->left is pointing at null
Step 10: root == NULL
the root is pointing at null
true
return [0, 0]
We backtrack to step 9 and move forward.
left = [0, 0]
right = robHelper(root->right)
root->right is pointing at 1
Step 11: root == NULL
the root is pointing at 1
false
pair<int, int> left, right
left = robHelper(root->left)
root->left is pointing at null
Step 12: root == NULL
the root is pointing at null
true
return [0, 0]
We backtrack to step 11 and move forward.
left = [0, 0]
right = robHelper(root->right)
Step 13: root == NULL
the root is pointing at null
true
return [0, 0]
We backtrack to step 12 and move forward.
left = [0, 0]
right = [0, 0]
rootExcluded = Math.max(left[0], left[1]) + Math.max(right[0], right[1])
= Math.max(0, 0) + Math.max(0, 0)
= 0
rootIncluded = root.val + left[1] + right[1]
= 1 + 0 + 0
= 1
return [rootIncluded, rootExcluded]
[1, 0]
We return to step 10 and continue.
Step 14: left = [0, 0]
right = [1, 0]
rootExcluded = Math.max(left[0], left[1]) + Math.max(right[0], right[1])
= Math.max(0, 0) + Math.max(1, 0)
= 1
rootIncluded = root.val + left[1] + right[1]
= 3 + 0 + 0
= 3
return [rootIncluded, rootExcluded]
[3, 1]
We backtrack to step 8, where the root is 3
Step 15: left = [2, 3]
right = [3, 1]
rootExcluded = Math.max(left[0], left[1]) + Math.max(right[0], right[1])
= Math.max(2, 3) + Math.max(3, 1)
= 3 + 3
= 6
rootIncluded = root.val + left[1] + right[1]
= 3 + 3 + 1
= 7
return [rootIncluded, rootExcluded]
[6, 7]
We backtrack to step 1 in the rob function and continue.
// func rob(root)
Step 16: return max(result[0], result[1])
max(6, 7)
We return the result as 7.